《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

请叫我雷锋

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》
小型无人直升机线性与非线性控制
作者：
Ioannis A. Raptis
Department of Electrical and Computer
Engineering
Georgia Institute of Technology

Kimon P. Valavanis
Department of Electrical and Computer
Engineering, and
Department of Computer Science
School of Engineering and Computer
Science
University of Denver
出版社：Springer
出版时间：2011年

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

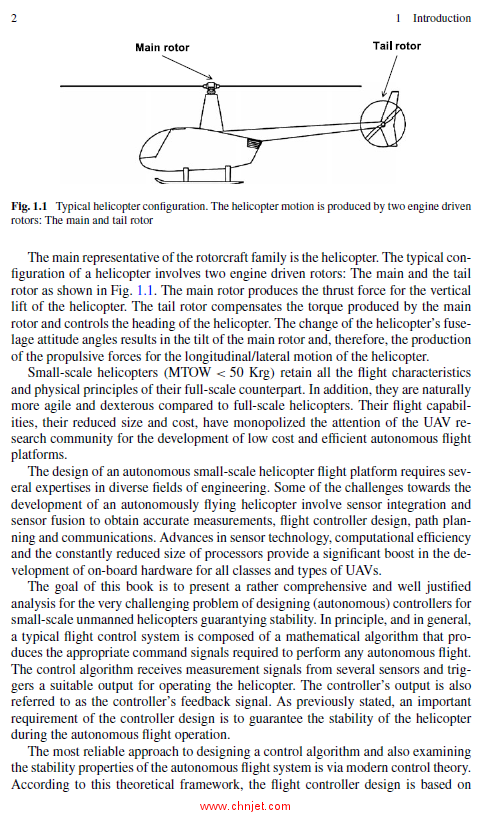
目录
1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1
1.1 Background Information . . . . . . . . . . . . . . . . . . . . . . . 1
1.2 TheMathematicalProblem . . . . . . . . . . . . . . . . . . . . . 4
1.3 ControllerDesigns . . . . . . . . . . . . . . . . . . . . . . . . . . 6
1.3.1 Linear Controller Design . . . . . . . . . . . . . . . . . . 7
1.3.2 Nonlinear Controller Design . . . . . . . . . . . . . . . . . 8
1.4 Outline of the Book . . . . . . . . . . . . . . . . . . . . . . . . . 9
2 Review of Linear and Nonlinear Controller Designs . . . . . . . . . . 13
2.1 Linear Controller Designs . . . . . . . . . . . . . . . . . . . . . . 14
2.2 Nonlinear Controller Design . . . . . . . . . . . . . . . . . . . . . 18
2.3 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 19
3 Helicopter Basic Equations of Motion . . . . . . . . . . . . . . . . . 21
3.1 Helicopter Equations of Motion . . . . . . . . . . . . . . . . . . . 21
3.2 Position and Orientation of the Helicopter . . . . . . . . . . . . . 25
3.2.1 Helicopter Position Dynamics . . . . . . . . . . . . . . . . 26
3.2.2 Helicopter Orientation Dynamics . . . . . . . . . . . . . . 27
3.3 Complete Helicopter Dynamics . . . . . . . . . . . . . . . . . . . 29
3.4 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 30
4 Simplified Rotor Dynamics . . . . . . . . . . . . . . . . . . . . . . . 31
4.1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 31
4.2 BladeMotion . . . . . . . . . . . . . . . . . . . . . . . . . . . . 32
4.3 Swashplate Mechanism . . . . . . . . . . . . . . . . . . . . . . . 34
4.4 Fundamental Rotor Aerodynamics . . . . . . . . . . . . . . . . . 36
4.5 Flapping Equations of Motion . . . . . . . . . . . . . . . . . . . . 40
4.6 RotorTip-Path-PlaneEquation . . . . . . . . . . . . . . . . . . . 42
4.7 FirstOrderTip-Path-PlaneEquations . . . . . . . . . . . . . . . . 43
4.8 MainRotorForces andMoments . . . . . . . . . . . . . . . . . . 44
4.9 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 45
xi
xii Contents
5 Frequency Domain System Identification . . . . . . . . . . . . . . . . 47
5.1 MathematicalModeling . . . . . . . . . . . . . . . . . . . . . . . 47
5.1.1 FirstPrinciplesModeling . . . . . . . . . . . . . . . . . . 48
5.1.2 SystemIdentificationModeling . . . . . . . . . . . . . . . 48
5.2 Frequency Domain System Identification . . . . . . . . . . . . . . 49
5.3 Advantages of the Frequency Domain Identification . . . . . . . . 50
5.4 Helicopter Identification Challenges . . . . . . . . . . . . . . . . . 51
5.5 Frequency Response and the Coherence Function . . . . . . . . . 51
5.6 The CIFER© Package . . . . . . . . . . . . . . . . . . . . . . . . 54
5.7 Time History Data and Excitation Inputs . . . . . . . . . . . . . . 55
5.8 Linearization of the Equations of Motion . . . . . . . . . . . . . . 56
5.9 Stability and Control Derivatives . . . . . . . . . . . . . . . . . . 58
5.10 Model Identification . . . . . . . . . . . . . . . . . . . . . . . . . 59
5.10.1 Experimental Platform . . . . . . . . . . . . . . . . . . . . 59
5.10.2 Parametrized State Space Model . . . . . . . . . . . . . . 61
5.10.3 IdentificationSetup . . . . . . . . . . . . . . . . . . . . . 63
5.10.4 TimeDomainValidation . . . . . . . . . . . . . . . . . . . 67
5.11 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 70
6 Linear Tracking Controller Design for Small-Scale Unmanned
Helicopters . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 73
6.1 Helicopter Linear Model . . . . . . . . . . . . . . . . . . . . . . . 73
6.2 Linear Controller Design Outline . . . . . . . . . . . . . . . . . . 75
6.3 Decomposing the System . . . . . . . . . . . . . . . . . . . . . . 77
6.4 Velocity and Heading Tracking Controller Design . . . . . . . . . 80
6.4.1 Lateral–Longitudinal Dynamics . . . . . . . . . . . . . . . 80
6.4.2 Yaw–Heave Dynamics . . . . . . . . . . . . . . . . . . . . 86
6.4.3 Stability of the Complete System Error Dynamics . . . . . 89
6.5 Position and Heading Tracking . . . . . . . . . . . . . . . . . . . 90
6.6 PIDControllerDesign . . . . . . . . . . . . . . . . . . . . . . . . 96
6.7 Experimental Results . . . . . . . . . . . . . . . . . . . . . . . . 98
6.8 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 102
7 Nonlinear Tracking Controller Design for Unmanned Helicopters . . 103
7.1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 103
7.2 Helicopter Nonlinear Model . . . . . . . . . . . . . . . . . . . . . 104
7.2.1 Rigid Body Dynamics . . . . . . . . . . . . . . . . . . . . 104
7.2.2 External Wrench Model . . . . . . . . . . . . . . . . . . . 105
7.2.3 Complete Rigid Body Dynamics . . . . . . . . . . . . . . 108
7.3 Translational Error Dynamics . . . . . . . . . . . . . . . . . . . . 108
7.4 Attitude Error Dynamics . . . . . . . . . . . . . . . . . . . . . . . 113
7.4.1 Yaw Error Dynamics . . . . . . . . . . . . . . . . . . . . . 113
7.4.2 Orientation Error Dynamics . . . . . . . . . . . . . . . . . 114
7.4.3 Angular Velocity Error Dynamics . . . . . . . . . . . . . . 114
Contents xiii
7.5 Stability of the Attitude Error Dynamics . . . . . . . . . . . . . . 115
7.6 Stability of the Translational Error Dynamics . . . . . . . . . . . . 121
7.7 NumericSimulationResults . . . . . . . . . . . . . . . . . . . . . 126
7.8 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 129
8 Time Domain Parameter Estimation and Applied Discrete
Nonlinear Control for Small-Scale Unmanned Helicopters . . . . . . 137
8.1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 137
8.2 Discrete System Dynamics . . . . . . . . . . . . . . . . . . . . . 138
8.3 Discrete Backstepping Algorithm . . . . . . . . . . . . . . . . . . 139
8.3.1 Angular Velocity Dynamics . . . . . . . . . . . . . . . . . 140
8.3.2 Translational Dynamics . . . . . . . . . . . . . . . . . . . 140
8.3.3 Yaw Dynamics . . . . . . . . . . . . . . . . . . . . . . . . 142
8.4 Parameter Estimation Using Recursive Least Squares . . . . . . . 144
8.5 Parametric Model . . . . . . . . . . . . . . . . . . . . . . . . . . 144
8.6 Experimental Results . . . . . . . . . . . . . . . . . . . . . . . . 145
8.6.1 Time History Data and Excitation Inputs . . . . . . . . . . 145
8.6.2 Validation . . . . . . . . . . . . . . . . . . . . . . . . . . 146
8.6.3 ControlDesign . . . . . . . . . . . . . . . . . . . . . . . . 146
8.7 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 149
9 Time Domain System Identification for Small-Scale Unmanned
Helicopters Using Fuzzy Models . . . . . . . . . . . . . . . . . . . . . 153
9.1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 153
9.2 Takagi–Sugeno Fuzzy Models . . . . . . . . . . . . . . . . . . . . 153
9.3 Proposed Takagi–Sugeno System for Helicopters . . . . . . . . . . 155
9.4 Experimental Results . . . . . . . . . . . . . . . . . . . . . . . . 156
9.4.1 Tunning of the Membership Function Parameters . . . . . . 156
9.4.2 Validation . . . . . . . . . . . . . . . . . . . . . . . . . . 157
10 Comparison Studies . . . . . . . . . . . . . . . . . . . . . . . . . . . 161
10.1 Summaryof theControllerDesigns . . . . . . . . . . . . . . . . . 161
10.2 Experimental Results . . . . . . . . . . . . . . . . . . . . . . . . 162
10.3 First Maneuver: Forward Flight . . . . . . . . . . . . . . . . . . . 162
10.4 Second Maneuver: Aggressive Forward Flight . . . . . . . . . . . 163
10.5 Third Maneuver: 8 Shaped Trajectory . . . . . . . . . . . . . . . . 170
10.6 Fourth Maneuver: Pirouette Trajectory . . . . . . . . . . . . . . . 171
10.7 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 175
11 Epilogue . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 181
11.1 Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 181
11.2 Advantages and Novelties of the Designs . . . . . . . . . . . . . . 182
11.3 TestingandImplementation . . . . . . . . . . . . . . . . . . . . . 183
11.4 Remarks . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 184
xiv Contents
Appendix Fundamentals of Backstepping Control . . . . . . . . . . . . 185
A.1 Integrator Backstepping . . . . . . . . . . . . . . . . . . . . . . . 186
A.2 Example of a Recursive Backstepping Design . . . . . . . . . . . 187
References . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 191

这本书出中文版了，对英文有难度的朋友可以购买中文版的阅读

《Linear and Nonlinear Control of Small-Scale Unmanned Helicopters》

专业书籍
下载地址：（回复后可见）

游客，如果您要查看本帖隐藏内容请回复

Ken

下載收藏,謝謝分享!

璀璨星空

谢谢分享。
来自: 微社区

hsjfdl

小型无人机

yxq123

谢谢分享

御锋

谢谢

GOOWOO

好书

大猴子

感谢

viviwan

谢谢分享

尚成

感谢